The local Reynolds number is then given by Re x = ρ Ux/ μ As the fluid flows past the long flat plate, the flow will become turbulent at a critical distance x cr downstream from the leading edge. For flow past a flat plate, the transition from laminar to turbulent begins when the critical Reynolds number (Re xcr) reaches 5×10 5. The boundary layer changes from laminar to turbulent at this point.

• Assuming A Transition Reynolds Number Of State

The Knudsen number (Kn) is a dimensionless number defined as the ratio of the molecular mean free path length to a representative physical length scale. This length scale could be, for example, the radius of a body in a fluid. The number is named after Danish physicist Martin Knudsen (1871–1949).

The Knudsen number helps determine whether statistical mechanics or the continuum mechanics formulation of fluid dynamics should be used to model a situation. If the Knudsen number is near or greater than one, the mean free path of a molecule is comparable to a length scale of the problem, and the continuum assumption of fluid mechanics is no longer a good approximation. In such cases, statistical methods should be used.

Definition[edit]

The Knudsen number is a dimensionless number defined as

${\displaystyle \mathrm{K}\mathrm{n}=\frac{\lambda }{L},}$

where

${\displaystyle \lambda }$ = mean free path [L¹],

${\displaystyle L}$ = representative physical length scale [L¹].

The representative length scale considered, ${\displaystyle L}$, may correspond to various physical traits of a system, but most commonly relates to a gap length over which thermal transport or mass transport occurs through a gas phase. This is the case in porous and granular materials, where the thermal transport through a gas phase depends highly on its pressure and the consequent mean free path of molecules in this phase.^[1] For a Boltzmann gas, the mean free path may be readily calculated, so that

${\displaystyle \mathrm{K}\mathrm{n}=\frac{k_{\text{B}}T}{\sqrt{2}\pi d^{2}pL},}$

where

${\displaystyle k_{\text{B}}}$ is the Boltzmann constant (1.3806504(24) × 10⁻²³ J/K in SI units) [M¹ L² T⁻² θ⁻¹],

${\displaystyle T}$ is the thermodynamic temperature [θ¹],

${\displaystyle d}$ is the particle hard-shell diameter [L¹],

${\displaystyle p}$ is the total pressure [M¹ L⁻¹ T⁻²].

For particle dynamics in the atmosphere, and assuming standard temperature and pressure, i.e. 25 °C and 1 atm, we have ${\displaystyle \lambda }$ ≈ 8×10⁻⁸ m (80 nm).

Relationship to Mach and Reynolds numbers in gases[edit]

The Knudsen number can be related to the Mach number and the Reynolds number.

Using the dynamic viscosity

${\displaystyle \mu =\frac{1}{2}\rho \overline{c}\lambda ,}$

with the average molecule speed (from Maxwell–Boltzmann distribution)

${\displaystyle \overline{c}=\sqrt{\frac{8k_{\text{B}}T}{\pi m}},}$

the mean free path is determined as follows:^[2]

${\displaystyle \lambda =\frac{\mu }{\rho }\sqrt{\frac{\pi m}{2k_{\text{B}}T}}.}$

Dividing through by L (some characteristic length), the Knudsen number is obtained:

${\displaystyle \mathrm{K}\mathrm{n}=\frac{\lambda }{L}=\frac{\mu }{\rho L}\sqrt{\frac{\pi m}{2k_{\text{B}}T}},}$

where

${\displaystyle \overline{c}}$ is the average molecular speed from the Maxwell–Boltzmann distribution [L¹ T⁻¹],

T is the thermodynamic temperature [θ¹],

μ is the dynamic viscosity [M¹ L⁻¹ T⁻¹],

m is the molecular mass [M¹],

k_B is the Boltzmann constant [M¹ L² T⁻² θ⁻¹],

ρ is the density [M¹ L⁻³].

The dimensionless Mach number can be written as

${\displaystyle \mathrm{M}\mathrm{a}=\frac{U_{\mathrm{\infty }}}{c_{\text{s}}},}$

where the speed of sound is given by

${\displaystyle c_{\text{s}}=\sqrt{\frac{\gamma RT}{M}}=\sqrt{\frac{\gamma k_{\text{B}}T}{m}},}$

where

U_∞ is the freestream speed [L¹ T⁻¹],

R is the Universal gas constant (in SI, 8.314 47215 J K⁻¹ mol⁻¹) [M¹ L² T⁻² θ⁻¹ mol⁻¹],

M is the molar mass [M¹ mol⁻¹],

${\displaystyle \gamma }$ is the ratio of specific heats [1].

The dimensionless Reynolds number can be written as

${\displaystyle \mathrm{R}\mathrm{e}=\frac{\rho U_{\mathrm{\infty }}L}{\mu }.}$

Dividing the Mach number by the Reynolds number:

${\displaystyle \frac{\mathrm{M}\mathrm{a}}{\mathrm{R}\mathrm{e}}=\frac{U_{\mathrm{\infty }}/c_{\text{s}}}{\rho U_{\mathrm{\infty }}L/\mu }=\frac{\mu }{\rho L{c}_{\text{s}}}=\frac{\mu }{\rho L\sqrt{\frac{\gamma k_{\text{B}}T}{m}}}=\frac{\mu }{\rho L}\sqrt{\frac{m}{\gamma k_{\text{B}}T}}}$

and by multiplying by ${\displaystyle \sqrt{\frac{\gamma \pi }{2}}}$ yields the Knudsen number:

${\displaystyle \frac{\mu }{\rho L}\sqrt{\frac{m}{\gamma k_{\text{B}}T}}\sqrt{\frac{\gamma \pi }{2}}=\frac{\mu }{\rho L}\sqrt{\frac{\pi m}{2k_{\text{B}}T}}=\mathrm{K}\mathrm{n}.}$

The Mach, Reynolds and Knudsen numbers are therefore related by

${\displaystyle \mathrm{K}\mathrm{n}=\frac{\mathrm{M}\mathrm{a}}{\mathrm{R}\mathrm{e}}\sqrt{\frac{\gamma \pi }{2}}.}$

Application[edit]

Problems with high Knudsen numbers include the calculation of the motion of a dust particle through the lower atmosphere and the motion of a satellite through the exosphere. One of the most widely used applications for the Knudsen number is in microfluidics and MEMS device design. Movements of fluids in situations with a high Knudsen number are said to exhibit Knudsen flow.

Airflow around an aircraft has a low Knudsen number, making it firmly in the realm of continuum mechanics. Using the Knudsen number an adjustment for Stokes' law can be used in the Cunningham correction factor, this is a drag force correction due to slip in small particles (i.e. d_p < 5 µm). The flow of water through a nozzle will usually be a situation with a low Knudsen number.^[3]

Mixtures of gases with different molecular masses can be partly separated by sending the mixture through small holes of a thin wall because the numbers of molecules that pass through a hole is proportional to the pressure of the gas and inversely proportional to its molecular mass. The technique has been used to separate isotopic mixtures, such as uranium, using porous membranes,^[4] It has also been successfully demonstrated for use in hydrogen production from water.^[5]

Assuming A Transition Reynolds Number Of State

One source says that Kn > 10 is a suitable criterion for distinguishing molecular flow from continuum flow.^[3]

See also[edit]

References[edit]

1. ^Dai; et al. (2016). 'Effective Thermal Conductivity of Submicron Powders: A Numerical Study'. Applied Mechanics and Materials. 846: 500–505. doi:10.4028/www.scientific.net/AMM.846.500.
2. ^Dai, W.; et al. (2017). 'Influence of gas pressure on the effective thermal conductivity of ceramic breeder pebble beds'. Fusion Engineering and Design. 118: 45–51. doi:10.1016/j.fusengdes.2017.03.073.
3. ^ ^abLaurendeau, Normand M. (2005). Statistical thermodynamics: fundamentals and applications. Cambridge University Press. p. 306. ISBN0-521-84635-8., Appendix N, page 434
4. ^Villani, S. (1976). Isotope Separation. Hinsdale, Ill.: American Nuclear Society.
5. ^Kogan, A. (1998). 'Direct solar thermal splitting of water and on-site separation of the products - II. Experimental feasibility study'. International Journal of Hydrogen Energy. Great Britain: Elsevier Science Ltd. 23 (2): 89–98. doi:10.1016/S0360-3199(97)00038-4.

• Cussler, E. L. (1997). Diffusion: Mass Transfer in Fluid Systems. Cambridge University Press. ISBN0-521-45078-0.

External links[edit]

The process of a laminar flow becoming turbulent is known as laminar–turbulent transition. The main parameter characterizing transition is the Reynolds number.

Transition is often described as a process proceeding through a series of stages. 'Transitional flow' can refer to transition in either direction, that is laminar–turbulent transitional or turbulent–laminar transitional flow.

The process applies to any fluid flow, and is most often used in the context of boundary layers.

• 2Transition stages in a boundary layer
  • 2.2Primary mode growth

History[edit]

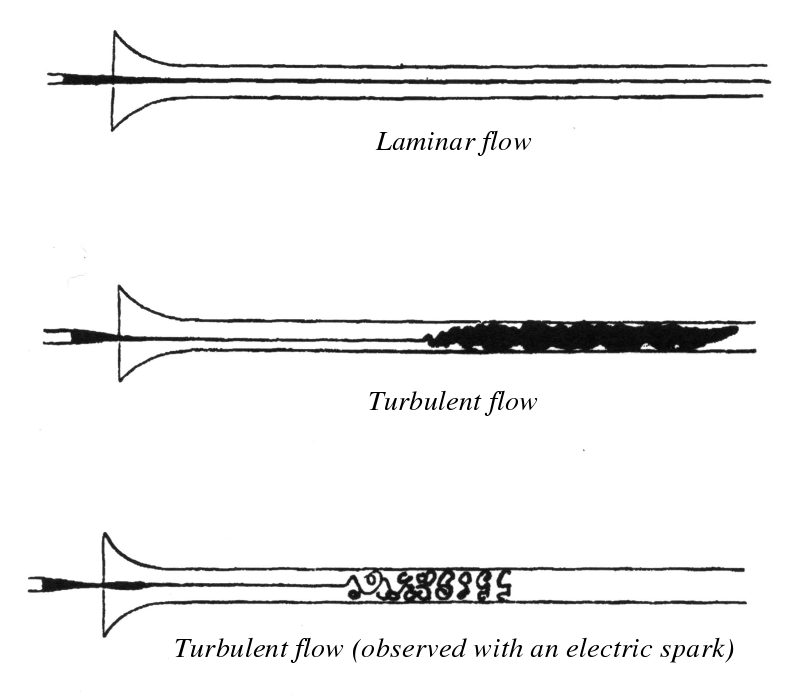
In 1883 Osborne Reynolds demonstrated the transition to turbulent flow in a classic experiment in which he examined the behaviour of water flow under different flow rates using a small jet of dyed water introduced into the centre of flow in a larger pipe.

The larger pipe was glass, so the behaviour of the layer of dyed flow could be observed, and at the end of this pipe was a flow-control valve used to vary the water velocity inside the tube. When the velocity was low, the dyed layer remained distinct through the entire length of the large tube. When the velocity was increased, the layer broke up at a given point and diffused throughout the fluid's cross-section. The point at which this happened was the transition point from laminar to turbulent flow. Reynolds identified the governing parameter for the onset of this effect, which was a dimensionless constant later called the Reynolds number.

Reynolds found that the transition occurred between Re = 2000 and 13000, depending on the smoothness of the entry conditions. When extreme care is taken, the transition can even happen with Re as high as 40000. On the other hand, Re = 2000 appears to be about the lowest value obtained at a rough entrance.^[1]

Reynolds' publications in fluid dynamics began in the early 1870s. His final theoretical model published in the mid-1890s is still the standard mathematical framework used today. Examples of titles from his more groundbreaking reports are:

Improvements in Apparatus for Obtaining Motive Power from Fluids and also for Raising or Forcing Fluids (1875)

An experimental investigation of the circumstances which determine whether the motion of water in parallel channels shall be direct or sinuous and of the law of resistance in parallel channels (1883)

On the dynamical theory of incompressible viscous fluids and the determination of the criterion (1895)

Transition stages in a boundary layer[edit]

A boundary layer can transition to turbulence through a number of paths. Which path is realized physically depends on the initial conditions such as initial disturbance amplitude and surface roughness. The level of understanding of each phase varies greatly, from near complete understanding of primary mode growth to a near-complete lack of understanding of bypass mechanisms.

Receptivity[edit]

The initial stage of the natural transition process is known as the Receptivity phase and consists of the transformation of environmental disturbances – both acoustic (sound) and vortical (turbulence) – into small perturbations within the boundary layer. The mechanisms by which these disturbances arise are varied and include freestream sound and/or turbulence interacting with surface curvature, shape discontinuities and surface roughness. These initial conditions are small, often unmeasurable perturbations to the basic state flow. From here, the growth (or decay) of these disturbances depends on the nature of the disturbance and the nature of the basic state. Acoustic disturbances tend to excite two-dimensional instabilities such as Tollmien–Schlichting waves (T-S waves), while vortical disturbances tend to lead to the growth of three-dimensional phenomena such as the crossflow instability.^[3]

Numerous experiments in recent decades have revealed that the extent of the amplification region, and hence the location of the transition point on the body surface, is strongly dependent not only upon the amplitude and/or the spectrum of external disturbances but also on their physical nature. Some of the disturbances easily penetrate into the boundary layer whilst others do not. Consequently, the concept of boundary layer transition is a complex one and still lacks a complete theoretical exposition.

Primary mode growth[edit]

If the initial, environmentally-generated disturbance is small enough, the next stage of the transition process is that of primary mode growth. In this stage, the initial disturbances grow (or decay) in a manner described by linear stability theory.^[4] The specific instabilities that are exhibited in reality depend on the geometry of the problem and the nature and amplitude of initial disturbances. Across a range of Reynolds numbers in a given flow configuration, the most amplified modes can and often do vary.

There are several major types of instability which commonly occur in boundary layers. In subsonic and early supersonic flows, the dominant two-dimensional instabilities are T-S waves. For flows in which a three-dimensional boundary layer develops such as a swept wing, the crossflow instability becomes important. For flows navigating concave surface curvature, Görtler vortices may become the dominant instability. Each instability has its own physical origins and its own set of control strategies - some of which are contraindicated by other instabilities – adding to the difficulty in controlling laminar-turbulent transition.

Simple harmonic boundary layer sound in the physics of transition to turbulence[edit]

Simple harmonic sound as a precipitating factor in the sudden transition from laminar to turbulent flow might be attributed to Elizabeth Barrett Browning. Her poem, Aurora Leigh (1856), revealed how musical notes (the pealing of a particular church bell), triggered wavering turbulence in the previously steady laminar-flow flames of street gaslights (“...gaslights tremble in the streets and squares”: Hair 2016). Her instantly acclaimed poem might have alerted scientists (e.g., Leconte 1859) to the influence of simple harmonic (SH) sound as a cause of turbulence. A contemporary flurry of scientific interest in this effect culminated in Sir John Tyndall (1867) deducing that specific SH sounds, directed perpendicular to the flow had waves that blended with similar SH waves created by friction along the boundaries of tubes, amplifying them and triggering the phenomenon of high-resistance turbulent flow. His interpretation re-surfaced over 100 years later (Hamilton 2015).

Tollmien (1931) and Schlichting (1929) proposed that friction (viscosity) along a smooth flat boundary, created SH boundary layer (BL) oscillations that gradually increased in amplitude until turbulence erupted. Although contemporary wind tunnels failed to confirm the theory, Schubauer and Skramstad (1943) created a refined wind tunnel that deadened the vibrations and sounds that might impinge on the wind tunnel flat plate flow studies. They confirmed the development of SH long-crested BL oscillations, the dynamic shear waves of transition to turbulence. They showed that specific SH fluttering vibrations induced electromagnetically into a BL ferromagnetic ribbon could amplify similar flow-induced SH BL flutter (BLF) waves, precipitating turbulence at much lower flow rates. Furthermore, certain other specific frequencies interfered with the development of the SH BLF waves, preserving laminar flow to higher flow rates.

An oscillation of a mass in a fluid is a vibration that creates a sound wave. SH BLF oscillations in boundary layer fluid along a flat plate must produce SH sound that reflects off the boundary perpendicular to the fluid laminae. In late transition, Schubauer and Skramstad found foci of amplification of BL oscillations, associated with bursts of noise (“turbulent spots”). Focal amplification of the transverse sound in late transition was associated with BL vortex formation.

The focal amplified sound of turbulent spots along a flat plate with high energy oscillation of molecules perpendicularly through the laminae, might suddenly cause localized freezing of laminar slip. The sudden braking of “frozen” spots of fluid would transfer resistance to the high resistance at the boundary, and might explain the head-over-heels BL vortices of late transition. Osborne Reynolds described similar turbulent spots during transition in water flow in cylinders (“flashes of turbulence,” 1883).

When many random vortices erupt as turbulence onsets, the generalized freezing of laminar slip (laminar interlocking) is associated with noise and a dramatic increase in resistance to flow. This might also explain the parabolic isovelocity profile of laminar flow abruptly changing to the flattened profile of turbulent flow – as laminar slip is replaced by laminar interlocking as turbulence erupts (Hamilton 2015).

Secondary instabilities[edit]

The primary modes themselves don't actually lead directly to breakdown, but instead lead to the formation of secondary instability mechanisms. As the primary modes grow and distort the mean flow, they begin to exhibit nonlinearities and linear theory no longer applies. Complicating the matter is the growing distortion of the mean flow, which can lead to inflection points in the velocity profile a situation shown by Lord Rayleigh to indicate absolute instability in a boundary layer. These secondary instabilities lead rapidly to breakdown. These secondary instabilities are often much higher in frequency than their linear precursors.

See also[edit]

References[edit]

1. ^Fung, Y. C. (1990). Biomechanics – Motion, flow, stress and growth. New York (USA): Springer-Verlag. p. 569.
2. ^Morkovin M. V., Reshotko E., Herbert T. 1994. 'Transition in open flow systems—a reassessment'. Bull. Am. Phys. Soc. 39:1882.
3. ^Saric W. S., Reed H. L., Kerschen E. J. 2002. 'Boundary-layer receptivity to freestream disturbances'. Annu. Rev. Fluid Mech. 34:291–319.
4. ^Mack L. M. 1984. 'Boundary-layer linear stability theory'. AGARD Rep. No. 709.
5. ^E. B. BROWNING, Aurora Leigh, Chapman and Hall, Book 8, lines 44–48 (1857).D. S. HAIR, Fresh Strange Music – Elizabeth Barrett Browning’s Language, McGill-Queens University Press, London, Ontario, 214–217 (2015).G. HAMILTON, Simple Harmonics, Aylmer Express, Aylmer, Ontario (2015).J. LECONTE, Phil. Mag., 15, 235-239 (1859 Klasse, 181–208 (1933).REYNOLDS Phil. Trans. Roy. Soc., London 174, 935–998 (1883).W. TOLLMIEN, Über die Enstehung der Turbulenz. 1. Mitteilung, Nachichten der Gesellschaft der Wissenshaften (1931).H. SCHLICHTING, Zur Enstehung der Turbulenz bei der Plattenströmung. Nachrichten der Gesellschaft der Wissenschaften – enshaften zu Göttingen, Mathematisch – Physikalische zu Göttingen, Mathematisch – Physikalische Klasse, 21–44 (1929).